| Physics and falling damage | Kinetic energy is the key | - | - | - |
| - | - | - | Dragon 88 | Dragon |
Physics and falling damage
Velocity's the key to understanding crash landings
by Arn Ashleigh Parker
| Defining the terms | - | - | - | The physics of falling damage |
| - | - | Falling Damage | - | - |
Holger thrust his sword into the giant's
thigh. The giant grunted; then he looked
down at Holger and drooled. Holger tried
to move away, but he was already dangerously
close to the edge of the cliff. He
turned back again in time to see the shadow
of the giant's body envelop his form. Then
he looked up and saw the giant raise one
enormous fist. If the blow didn't kill him
outright, surely it would propel Holger over
the edge of the cliff. In what would perhaps
be his last conscious decision, Holger decided
to take his chances with the cliff. He
spun around and muttered a silent prayer
as he stepped outward -- and down. . . .
| The following terms are used in the physics falling damage article
and the accompanying
equations article. You don?t have to study these definitions at length; just refer to them if you need assistance in deciphering an equation. |
- |
| V |
The speed of a body in the z, or ?down,? direction. |
| V |
The velocity in one dimension. |
| V |
The initial velocity of a body. |
| a g |
Acceleration due to gravity. |
| a x |
Acceleration in one dimension. |
|
Z |
Distance fallen. |
| X |
Distance moved in one dimension. |
| d |
The number of six-sided dice of damage caused by the fall. |
| t |
The time elapsed at a given instant. |
| t |
The time when movement began. |
|
P |
The potential energy of a system. |
| K |
The kinetic energy of a body. |
| m |
The mass of a body. |
| k |
A constant. |
| <> |
Indicates a change in the quantity that follows it (pronounced ?delta?). |
| <> |
A line over a letter or value indicates the average of the value in
question (in this case, average velocity). |
What happens to Holger now?
Can he survive the fall, or would he have been
better off letting the giant put him out of his
misery? The answer to that question depends
on a couple of important factors --
first, the height of the cliff, and second, the
system for computing falling damage that is
used in Holger's world.
The standard methods for determining
falling damage in the AD&D® game
can be,
extremely confusing. The system from the
PH calls for 1d6 of damage
per 10 feet fallen, to a maximum of 20d6 for
a 200-foot fall. A revised system, described
in issue #70 of DRAGON® Magazine by
Frank Mentzer, requires a cumulative 1d6
of damage per 10 feet fallen, to a max.
of 20d6 at just under 60 feet -- therefore,
the character takes 1d6 damage for 10?, 1d6
+ 2d6 (=3d6) for 20?, 1d6 + 2d6 + 3d6 (=6d6)
for 30? ? and the damage adds up in a
hurry. (Editor?s note: According to Frank?s
article, this was the system that AD&D
game designer E. Gary Gygax intended to
be part of the rules, but the pertinent
passage
in the PH was edited so
that the meaning of Gary's original rule was
altered.) Unfortunately, neither of these
systems agrees with the laws of physics
governing falling bodies.
The purpose of this article is to offer an
alternative system for falling damage that
pays special attention to the physical laws of
the real world. This proposed system is
based on the laws of velocity. Before launching
into an explanation of the system, however,
we'd best get our vocabulary straight,
and define some of the terms we need to
use.
The first equation given in the short
article on the facing page defines velocity as
follows:
The average velocity in one dimension ![]() ,
,
equals the distance moved ![]()
divided by the time elapsed ![]()
.
For example, if you run 100 yards in 10
seconds, then you have run at an average
speed of 10 yards per second. If you run at
that speed in a specified direction -- say,
down a football field -- then you have run
at an average velocity of 10 yards per second
in the direction of the football field?s
end zone. In short, velocity is speed with a
direction attached.
The example above illustrates average
velocity. In physics, however, instantaneous
velocity is far more useful. If your velocity
changes while you run down the field ?
you jog for 5 seconds and sprint the rest of
the way -- then your average velocity tells
us nothing about your speed at any given
moment. Instantaneous velocity describes
this. If you'd like to see the scientific definition
of instantaneous velocity, refer to the
equations article.
Because average and instantaneous velocities
can differ, we know that acceleration
can be a factor: if you start slow and end
fast, you've accelerated. Like velocity,
acceleration can be measured as an average
quantity or an instantaneous one. Equations
for acceleration are also included in
the accompanying article. We?ll draw from
these equations later, but you don?t need to
study them now.
The physics of falling damage
Using physics to describe falling damage
is not an open-and-shut proposition, because
no definitive method exists. Hit
points reflect a fantasy situation where
injury is quantified. But in the real world,
we cannot quantify injury; we can only
measure it in qualitative terms. For example,
let's say two cars collide head-on.
Driver A ends up with a fractured wrist.
Driver B receives a concussion, a broken
arm, and a back injury. How much worse
off is Driver B than Driver A? Three times?
Ten times? A hundred times? The point is
this: We cannot describe real injury in
quantitative terms -- a broken arm does
not mean one-eighth dead, for example. We
can only describe real injury in qualitative
terms; the back injury was severe, the fractured
wrist comparatively minor.
But hit points are a quantitative measurement.
Therefore, we must make an assumption
as to what quantitative property in
physics can best relate to the calculation of
falling damage. I believe that the property
in question is velocity, and I believe that the
relationship between velocity and falling
damage is linear. If a character hits the
ground at speed x, then he should take x
points of damage. If he hits the ground at
speed 2x, then he should take 2x points of
damage, and so on.
If we accept this assumption, all we have
to do is determine the velocity at which a
character hits the ground if he falls from a
given height. Equations 1 and 5 from the
equations article can get us started. Together,
they allow us to derive the following
table, which provides the instantaneous
velocity at the time of impact for specific
distances fallen.
Table I
| Time (sec.) | Instantaneous
velocity (ft./sec.) |
Distance
(ft.) |
| 1 | 32 | 16 |
| 2 | 64 | 64 |
| 3 | 96 | 144 |
| 4 | 128 | 256 |
| 5 | 160 | 400 |
| 6 | 192 | 576 |
Unfortunately, Table I does not include
the effects of air resistance. As a person
falls, the air retards his acceleration. As
time increases, the person?s velocity approaches
a constant value, eventually reaching
a point at which he keeps falling at the
same speed. This value is called terminal
velocity -- the maximum velocity of a
falling body. Air friction acts as a balancing
force, eventually stopping the acceleration
caused by gravity.
Terminal velocity is important to understand,
because that should be the velocity at
which maximum damage occurs. A person
may keep falling, but if his velocity no
longer increases, the damage he incurs
shouldn't increase either.
The following table is extracted from the
book Skydiving by Bud Sellick. The table
gives the actual distance that a 200-pound
person falls during a free fall of a certain
duration, if the person falls from an initial
height of 2,200 feet.
Table II
| Time
(sec.) |
Avg. velocity for
each sec (ft./sec.) |
Distance
(ft.) |
| 1 | 16 | 16 |
| 2 | 46 | 62 |
| 3 | 76 | 138 |
| 4 | 104 | 242 |
| 5 | 124 | 366 |
| 6 | 138 | 504 |
| 12 | 174* | 1500 |
* -- terminal velocity
If we compare Tables I and II, we see
that air resistance does have some effect on
velocity, which is reflected by the distance
fallen. According to Sellick, terminal velocity
varies depending on the height from
which a person falls, and it takes anywhere
from 12 to 14 seconds to reach terminal
velocity in a skydiving free fall. If the person
falls from 30,000 feet, his terminal
velocity is 235 ft./sec. If he falls from 1,000
feet, his terminal velocity is 160 ft./sec. If
we compare these figures to the terminal
velocity in Table II (for a fall of 2,200 feet),
we see that the terminal velocity is indeed
lower when the person falls from a lower
elevation.
But few characters in the AD&D game
fall from 30,000 feet, or even 1,000 feet. In
order to make the physics falling damage
system work, we have to determine a terminal
velocity that is appropriate to the common
heights in game play.
Let's look at Table II. After 5 seconds of
falling, the increase in average velocity
(from the 5th to the 6th second, and presumably
from second to second thereafter)
seems insignificant. How do we decide what
is insignificant? I propose that any increase
in the average velocity that is less than the
average velocity during the first second of
the fall is insignificant for our purposes. In
Table II, the average velocity for the first
second of the fall is 16 ft./sec. The increase
from the 5th to the 6th second is only 14 ft./
sec. (138 minus 124). Therefore, I believe
the increase in velocity after the 5th second
is insignificant.
* * * * *
After the publication of the
lengthy article
"Physics and Falling Damage"
in issue #88, I
feel compelled to voice a
concern.
When I first opened my Players
Handbook,
one thing was clear to me
-- this was a game, not
a simulation. Characters
could do superhuman
feats of strength and magic.
Also, the combat
system revolved around one-minute
intervals of
time. It was very unrealistic
-- it was a game.
However, in the last few years
DRAGON
Magazine has time and again
presented "realistic
" studies about combat, weather,
etc. In fact,
the general attitude of the
gaming public has
shifted toward simulations.
The most extreme example of
this attempt at
realism came in the form
of the aforementioned
article. 6 pages were devoted
to a complicated
detailing of a falling damage
system. "Scientific"
facts and theories were presented
to give a realistic
simulation of the effects
of a falling body in a
gravity field. It was very
interesting, very
lengthy, and very un-needed.
Mr. Gygax himself states on
page 9 of the
DMG that AD&D
is a game, not a simulation.
<APPROACHES
TO PLAYING ADVANCED DUNGEONS & DRAGONS>
Further, he says that any
attempt at realism
would be an "absurd effort."
Also, he writes that
a realistic simulation in
the realm of make-believe
?can be deemed only a dismal
failure?; and also
that readers who seek realism
?must search
elsewhere.?
Often, this magazine presents
articles trying to
explain rules (in the AD&D
game) in realistic
terms. What a waste of energy,
time, and space!
To try to add realistic changes
into an inherently
unrealistic game would bring
about its collapse.
Many times you have tried
to explain, in realistic
terms, why certain classes
can or cannot use
certain weapons. This is
un-needed, for those
restrictions were made to
keep the game in balance
and to aid in role-playing.
Instead of explaining
why or why not certain weapons
can be
used, for instance, maybe
the space could be used
to list new weapons, or ways
players can maximize
available weapon use.
In conclusion, I would like
to say (in my opinion,
of course) that the downfall
of the AD&D
game may not come from an
outside agent, but
from within the gaming world.
In the quest for
quasi-realism, the game may
be greatly altered or
even forgotten. This would
be a great loss.
Jeff Martin
Marion, Ill.
* * * *
I am writing in response to
the article ?Physics
and Falling Damage? (#88).
According to that
article, "falls of between
2 and 5 feet, including
falls from horseback, should
cause 1d6 of damage.
" I think that?s ridiculous.
That means that
the average first-level character
(who has about 6
hit points) who falls off
a horse seven times (at the
most) would be into negative
hit points. Maybe
it?s unlikely that someone
will fall off a horse
seven times, but it might
be while he?s just learning
to ride, or it doesn?t even
have to be off a
horse; he just has to fall
from between 2-5 feet.
Also, the article says, "For
falls of 2 feet or
less, only 1 point of damage
is incurred.? Only 1
point of damage !! That means
that when you
trip, you take a point of
damage. (When you trip,
most of your body travels
2 feet or more before
hitting the ground.)
I, for one, think that the
system presented in
the article is a waste when
there is an easier and
better
system in the Players Handbook.
Jim Tuttle
Millbury, Mass.
(Dragon #90)
* * * *
I read with interest Arn Ashleigh
Parker's
article concerning falling
damage in DRAGON <PH> <WSG>
#88. It appeared well organized
and showed
evidence of a lot of hard
work. That's what makes
it painful for me to point
out the fatal error in his
system. He is correct to
assume that velocity is
the most important factor
in calculating damage.
However, the relationship
between velocity and
falling damage is not linear,
but geometric.
According to the ballistics
tables in the Speer
Reloading Manual Number
Ten for Rifle and
Pistol, the
energy generated by a bullet increases
proportionally to the square
of the increase in the
velocity. Thus, an object
moving twice as fast will
generate 4 times as much
energy, and an object
moving 3 times as fast will
generate 9 times as
much energy.
As a graphic example, a person?s
nose hitting a
brick wall 30 times at a
velocity of 1 mph (about
1.5 feet per second) should
not incur as much
damage as the same nose hitting
once at 30 mph.
Steven
Winter has, in his rebuttal article,
picked
up on this error, and on one other --
namely,
that kinetic energy is what does the
damage.
A soft, falling body is unable to transfer
all
of its kinetic energy to the ground. It is the
energy
not transferred which does the damage to
the
body.
Jay D. Glithero
Bensalem, Pa.
(Dragon #90)
* * * *
I regret
that you have relegated the fundamental
rules
governing the universe to a matter of
opinion
in the pages of DRAGON #88. While
Steve
Winter's rebuttal does much to mitigate my
horror,
I still feel the need to comment on Arn
Ashleigh
Parker?s article about falling damage.
Mr. Parker presents a cogent
and physically
valid argument for kinetic
energy determining
injury from a fall, only
to arbitrarily dismiss the
conclusion because it doesn?t
sound right. We
have precious few physical
laws and ? despite his
claim to the contrary ? one
of these does necessitate
kinetic energy having a direct
effect on falling
damage. It is the often-quoted
Law of Conservation
of Matter and Energy, which
says in part
?energy is neither created
nor destroyed.? The
direct consequence of this
law is that all factors of
the kinetic energy equation
(not just the square
root of one factor) come
into play.
Mr. Parker's reply on intuition
and what sounds
right is not science.
It is handwaving and quakery.
Furthermore, the lack of
professionalism
displayed by DRAGON
Magazine in printing
such pseudo-science is disturbing.
In closing, let me say that
Steve Winter?s
rebuttal was eloquent and
meticulously correct as
far as he took it. There
remains, however, an
open niche for a falling
damage system based on
a given amount of damage
per unit of distance
fallen (like the Players
Handbook method), but
which incorporates a few
other factors. There is
no current provision for
good or poor landings,
no weight factor (mass is
every bit as important
as the squared velocity),
short falls are entirely
too lethal for zero- and
first-level characters, and
long falls are much too safe
for high-level adventurers.
Each of these points must
be addressed in
any viable alternative.
Jonathan Heiles
Pleasant Valley, N. Y.
(Dragon #90)
* * * *
In issue #88, I read an article
called ?Physics
and Falling Damage.? In this
article is a new
system for falling damage
which prescribes 1 hp
of damage for a 2? or less
fall, and a full d6 of
damage for falls of 2? to
5? and falls from a
horse. If someone took 1-6
points of damage
every time he or she fell
off a horse, there
wouldn?t be many bareback
riders in the circus,
would there? What about little
rich kids, or
nobles? kids with ponies?
I think that people who fall
2? should be inactive
for 4 or 5 segments, and
those who fall off
horses should take 1-3 points,
or thereabouts.
Ted Van Horn
Silver
Spring, Md.
* * * *
I think both Arn Ashleigh
Parker and Steven
Winter missed the point in
their articles on falling
damage (#88). First and foremost,
the damage
inflicted on any object via
falling occurs because
of the force it is subjected
to, not the velocity it
has prior to impact. It is
the sudden compression
of body tissue that counts.
If someone fell 100 feet
into a very large pile of
feathers, the same velocity
and kinetic energy would
be achieved during
the fall, as if he had fallen
on concrete, but with a
distinctly different outcome.
Force, according to Mr. Newton,
is equal to
the product of an object's
mass and its acceleration.
Because mass is constant,
the impact force
on an object is due to the
acceleration, which is
the rate at which velocity
changes with respect to
time.
Because this change of velocity is negative
when an object hits the ground,
we have a negative
acceleration, or deceleration.
The reason a man falling into
feathers fares
better than one falling on
concrete is that the
feathers “give” a little,
allowing the deceleration
to occur over a longer time
for the same change
in velocity, assuming each
man falls from the
same height. The deceleration
is less, and so is
the force of impact.
Since damage is usually assessed
on falls to
hard surfaces, an assumption
can be made which
will allow us to find a suitable
parameter for
damage. Because neither the
falling individual
nor the Earth is very elastic,
deceleration occurs
over a very short period
of time (small fraction of
a second), which is largely
independent of impact
velocity. In other words,
it is safe to assume that
whether a body hits the ground
at 10 feet a second
or 200 feet a second makes
little difference in
terms of how long it takes
that body to come to a
complete stop.
The happy implication of this
assumption is
that only the change in velocity
affects the force of
impact, and the degree of
damage must be proportional
to that velocity change.
Mr. Parker is
correct in his assertion
that damage is proportional
to impact velocity, but for
different reasons.
Nevertheless, his system
of saving throws seems a
bit too complicated; why
not reduce the figure of
20d6 as the maximum damage
allowance for
falling? This scaling-down
would yield a new
system that is playable,
while retaining damage
figures in keeping with falling
distance at most
heights.
Of course, the ultimate in
realistic assessment
of falling damage would have
to take into account
the fact that a falling person
absorbs damage in
unequal proportions to the
forces involved, unlike
falling objects which are
inanimate. A falling
branch absorbs twice as much
damage if the
impact velocity is doubled,
but a falling human
may suffer injury to a vital
organ or system that
he would not have suffered
at the original impact
velocity. The difference
in assessed hit points
upon doubling velocity might
be a factor of 3 or
10, rather than 2. So my
final impression is that,
naive as it might be, the
old system works fine.
Since the number of factors
that must be incorporated
to make any new system totally
realistic is
unworkable, why not leave
well enough alone?
David N. Moolten
Philadelphia, Pa.
* * * *
I have no idea whether velocity
is right, or if
kinetic energy really is
the key, but I do know
that both articles on falling
damage (issue #88)
overlooked something important.
The basis of the
subject is damage ? in other
words, hit points.
The explanation on p. 82
of the DMG basically
says that hit points are
made up of 1) physical
ability to withstand damage,
2) combat skill, 3)
?6th sense,? and 4) divine
protection. In falling
damage probably only the
physical ability and
divine protection would count.
Personally, I tend
to think hit points would
be made up more of
combat skill than anything
else. This is backed up
by the fighter?s greater
amount of hit points.
My point is that a first level
character should
take a fall almost as well
as a fifth, and a magicuser
as well as a ranger. So the
only answer is
damage based on a percentile
system. Is there a
reason why this hasn?t been
thought of before?
Mark Herman
Waterloo, Iowa
* *
* *
I?ve
been reading about all the new systems for
falling damage, and rebuttals
to them, for some
months now, and one thing
that struck me was
that everybody seems to
have forgotten the point
of the argument.
Does anybody else remember
the story about
the high-level fighter who
took a swan dive off a
cliff, picked himself up,
dusted himself off, and
then proceeded to slice
and dice? The point was
the falling damage system
does not do enough
damage to high level characters.
Since hit points are supposedly
a reflection of a
character?s ability to avoid
damage in combat due
to skill, and since falling
off a cliff seems to have
little to do with lighting,
I suggest a method of
determining falling damage
that does not rely on
a fixed scale of HP damage
per given distance
fallen. Thus, these tables:
If SAVE is made
| Distance | % of HP | K.O. | Kill |
| 10' | 0-40 | 5% | 1% |
| 20' | 5-50 | 15% | 3% |
| 30' | 10-60 | 25% | 6% |
| 40' | 15-70 | 35% | 10% |
| 50' | 20-80 | 45% | 15% |
| 60' | 25-90 | 55% | 21% |
| 70' | 30-99 | 65% | 28% |
| 80' | 40-99 | 75% | 36% |
| 90' | 50-99 | 85% | 45% |
| 100' | 60-99 | 95% | 55% |
If SAVE is NOT made
| Distance | % of hp | K.O. | Kill |
| 10' | 5-60 | 15% | 5% |
| 20' | 10-70 | 25% | 10% |
| 30' | 15-80 | 35% | 15% |
| 40' | 20-90 | 50% | 20% |
| 50' | 25-99 | 65% | 25% |
| 60' | 35-99 | 80% | 30% |
| 70' | 45-99 | 95% | 40% |
| 80' | 55-99 | 99% | 50% |
| 90' | 65-99 | 99% | 60% |
| 100' | 75-99 | 99% | 75% |
% of hp = The percentage of
hit points lost
(from current or total values,
at DM option);
a result of 0% means the
loss of 1 hit point.
K.O. = The chance that the
character is
knocked unconscious (brought
to zero hp) by
the fall.
Kill = The chance that the
character is killed
outright by the fall.
First, the character rolls
a save (I recommend a
save vs. paralyzation, with
a bonus of +1 per 3½
points of dexterity). Then,
roll d% and compare
it to the K.O. figure for
the distance in question
on the appropriate table.
If the result is greater
than the K.O. figure, the
character is not
knocked unconscious and
will take damage in the
range given. If the result
is equal to or less than
the K.O. figure, roll d%
again and compare that
result to the Kill figure.
If this second roll is equal
to or less than the Kill
figure, the character is
dead; otherwise, he is knocked
out and brought
to zero hit points.
This method insures that
while a high-level
character may not necessarily
die from a long fall,
he will take a considerable
amount of damage.
Since the table provides
a fair chance of survival,
DMs using it may want to
apply a penalty to the
save for low-level characters,
and an increase in
the percentage of damage
taken.
I have not extended the table
beyond 100 feet
for this reason: If any
character survives a fall of
more than 100 feet, some
form of DIVINE INTERVENTION must be involved.
The simplest way to extend
the table, if desired, is to increase the Kill
figure by 5% for each additional
10 feet.
Finally, any character taking
more than 50% of
his hit points in damage
from a fall will be
stunned for a number of
melee rounds equal to
the tens of feet fallen.
Any character knocked
unconscious remains so (unless
aided) for a
number of turns equal to
the tens of feet fallen.
Characters who are killed
outright just lie there.
It works for me.
William Huish
Las Vegas, Nev.
Dragon #93
* * * *
After reading William Huish's
letter on falling
damage (issue #93), I?m
glad to see that there are
still DMs who wish to keep
the
game accurate but
simple. I find his table
works very well, except
one item in taking a fall
is not there ? injuries
such as broken bones. When
a body falls from
any height and impacts with
a solid object there is
a chance for broken bones.
The percentage chance for
broken bones is
controlled by two factors,
the height of the fall
and whether the character
is in control of the fall.
Having a controlled fall
is being able to land on
your feet and roll or find
some other way to
absorb the shock. Controlling
a fall involves
whether the fall was taken
willingly and if any
obstacles were struck before
hitting the ground.
% chance of broken bones
| Distance | Controlled | Uncontrolled |
| 10' | 5% | 10% |
| 20' | 10 | 25 |
| 30' | 15 | 40 |
| 40' | 20 | 55 |
| 50' | 30 | 70 |
| 60' | 40 | 80 |
| 70' | 50 | 90 |
| 80' | 60 | 95 |
| 90' | 70 | 98 |
| 100' | 80 | 100 |
Monks may subtract 2% per level because
of
their training; thieves can subtract 1%
per level.
Any character who is heavily encumbered
must
add 15% due to the fact that they are
unable to
roll to absorb the damage.
The bones broken most often in a controlled
fall will be in the legs. With an uncontrolled
fall,
the break may occur anywhere. With a fall
from
over 20 feet the DM may wish to include
a
chance for head concussion
This letter is not meant to criticize Mr.
Huish.
I have found his tables the most useful
way of
determining fall damage and offer this
as an
enhancement. I, like many DMs, am looking
for
ways to make the game accurate, but most
of all
easy to play.
Calvin V. Jestice
Cincinnati, Ohio
Dragon #95
* *
* * *
Scientific facts behind the
system
The equations in this article give structure
The equation for instantaneous velocity
Average acceleration is defined as:
Instantaneous acceleration equals:
With the equations above, we can proceed
acceleration due to gravity, ag), the average
We can rearrange the equation above if we
Now, for a constant acceleration only, the
The position of the falling person (if the
By substituting the right side of equation 2
If we solve equation 3 for Vz and plug the
By solving equation 4 for z, we can find the
The value of equation 5 is this: we can find
If this value for t is plugged into equation 5,
If we multiply both sides of equation 6 by
Solving equation 7 for Vz gives us:
It is equation 8, referred to several times in
-
Equation #8 has a more severe mistake. It
The equation for finding the number of dice of
It is true that this has no significance when
the
Dan Redder
We conveyed Dan's observation to Arn
Arn also pointed out a detail that Dan didn’t
The error in equation #2 is relatively minor,
|
This definition of significance is acceptable
from both the standpoints of physics
and gameplay. Physicists are very willing to
approximate data when, as is the case here,
the increase in the average velocity is negligible
when compared to the average velocity.
This is especially so considering that
very few characters in an AD&D game
ever
fall from distances where the terminal velocity
varies less than 1 ft./sec. (starting at
about 1,500 feet).
Since the increase in average velocity
from the 5th to the 6th second is insignificant,
shall we say that the distance fallen
after 5 seconds ? 366 feet ? is where
terminal velocity occurs in the game? If
most adventurers fell from an elevation of
2,200 feet like the skydivers from Table II,
the answer would be yes. But most characters
fall from a much lower elevation. And
velocity increases more slowly at lower
elevations, because the air is heavier. Therefore,
the terminal velocity for our purposes
will be reached after a significantly shorter
distance has been fallen; 366 feet is still too
high.
A glance at Table II reveals that the
increase in average velocity from the end of
the 4th to the end of the 5th second is only
20 ft./sec. (124 minus 104). We know the
table is based on a fall from 2,200 feet ? an
elevation far higher than that most adventurers
would encounter. Therefore, it is not
unreasonable to assume that the velocity
difference for the 4th and 5th seconds of an
adventurer?s fall is less than 16 ft./sec., and
thus is an insignificant amount. We can also
assume that the terminal velocity of an
adventurer?s free fall, therefore, occurs at
about the 4th second of the fall. Looking at
Tables I and II, we can see that the character
would have fallen about 250 feet at this
point in time.
It's good that our approximation of where
terminal velocity (and hence, maximum
damage) occurs is so near 200 feet. Gamers
who are used to having maximum damage
occur at 200 feet (as per the Players Handbook)
won?t have to alter their conceptions
much to accommodate the physics falling
damage system.
I propose that we set 256 feet as the exact
distance at which terminal velocity is
reached. This distance is as accurate as any
other near 250 feet, and the number 256
makes the resultant equation in the physics
falling damage system easier to use and
remember.
With all the above points in mind, a brief
examination of the two current systems,
plus another common proposal, should yield
a good understanding of why those systems
don?t work ? and why a system based on
velocity will.
1d6 per 10 feet fallen
This system indicates that damage increases
linearly with the distance fallen: 1d6
for 10?, 2d6 for 20?, 3d6 for 30?, etc. Max.
damage (terminal velocity) is reached
at 200?, when the victim takes 20d6 points
of damage. The maximum damage point is
not too bad, but the damage taken before
that should not increase linearly with the
distance fallen. If we accept that velocity
relates directly to damage, the damage
should reflect the speed of the victim when
he hits the ground. As we can see from
Tables I and II, velocity does not increase
the same way distance does: speed increases
linearly, while distance increases geometrically.
In other words, distance increases
much faster than velocity. Therefore, if a
character takes 2d6 of damage in a fall from
20 feet, he should not take 4d6 in a fall from
40 feet; he should take less than 4d6.
1d6 cumulative per 10 feet fallen
In every sense, this system is worse than
the previous one. Instead of terminal velocity
being reached at 250? or even 200?, it is
attained at approximately 60?! Furthermore,
damage is 1d6 at 10?, 3d6 at 20?,
6d6 at 30? ? a geometric progression. This
directly opposes the real relationship between
distance and velocity, which is a
geometric retrogression.
Why kinetic energy isn't the answer
There are several principles on which
falling damage can be based. As I have
already stated , I believe the appropriate
principle is velocity. However, the energy
involved in a fall, particularly kinetic energy,
may also seem appropriate ? at first,
anyway. Is there a direct relationship between
kinetic energy and falling damage? I
intend to show that there is not, but before
that, we must clarify what the energy in a
fall is all about.
We will discuss two kinds of energy:
kinetic and potential. Kinetic energy is the
energy of motion. When a person is falling,
he has a certain amount of kinetic energy.
Potential energy is energy that is stored in a
system and cannot be attributed to any
particular object.
A person does work when he walks up a
hill ? work in the sense of physically opposing
a given force. The amount of work
he does equals the potential energy (P),
calculated by the equation P = magz.
The equation is read as ?Potential energy
equals mass times acceleration due to gravity
times the height above the earth.? This
assumes that at the surface of the earth
potential energy is zero, which is okay for
our discussion.
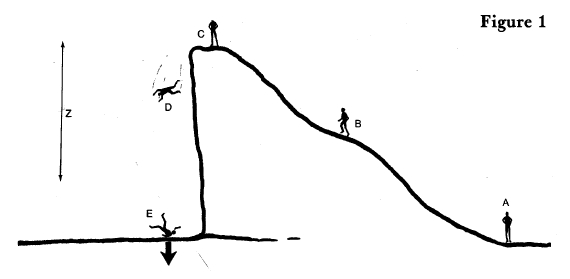
Figure 1 illustrates the physics of kinetic
and potential energies in a fall. At point A,
the person begins to climb the hill. Potential
energy equals zero. At point B, the person
uses "work" to walk up the hill. This
?work? gives the system (which includes
everything in the diagram) a potential energy
of magz when the person is at point C.
At point D, the total energy of the system
involves both some potential energy and
some kinetic energy; the former is present
in the system, while the latter has been
imparted to the falling person. Just before
point E, when the person is about to smash
into the earth, all of the energy in the system
is kinetic energy, all of which is contained
in the person?s body. When the
person hits the ground, he loses all of his
kinetic energy to the earth ? and the earth
moves a minuscule amount in the direction
indicated by the arrow.
Now that we understand the basics of
kinetic and potential energy (right?), we can
examine the relationship between kinetic
energy and falling damage. If kinetic energy
and falling damage were directly related, we
should be able to illustrate how kinetic
energy can be used to calculate the damage
from a fall.
If we accept that the transfer of kinetic
energy from the person to the earth is the
direct cause of falling damage, then we need
only use the implied linear relationship
between the two to set up our equation:
½mVz2 = magz = kd
Essentially, the equation reads ?Kinetic
energy equals potential energy, which is also
equal to a constant (k) times the dice of
damage sustained (d). This illustrates the
linear relationship between the distance
fallen (z) and the dice of damage sustained
in the fall (d) ? the original
system in the
PH!
But everything above is based on the
assumption that a linear relationship exists
between falling damage and kinetic energy.
No physical law exists that says kinetic
energy is the direct cause of physical injury.
We know that there is some relationship
between the two -- because the more kinetic
energy a person transfers to the earth,
the greater his injuries are. But no law
states that this relationship is linear, or that
all the factors involved in kinetic energy
relate to the injury. It may be, then, that
some part of kinetic energy relates linearly
to falling damage. Since no formula exists
to tell us what part this might be, we have
to use our intuition to determine the crucial
property.
Once again, we must ask ourselves, What
property could we reasonably assume to
have a linear relationship to falling damage?
A suitable answer, as I have said
before, would be velocity. It just seems right
that if a person takes d amount of damage
after falling at speed x, then that person
should take 2d of damage if he hits the
ground falling at a speed that?s twice as fast.
Some variation would exist, of course, but
that?s why we use six-sided dice to determine
falling damage, instead of just assigning
a number of hit points lost.
The problem with using kinetic energy to
determine damage is this: kinetic energy is
a function of the square of velocity. Everyday
physics (the classical mechanics) is very
much intuitive. It does not make sense that
the square of velocity linearly relates to
falling damage; it does make sense that
velocity itself directly relates to damage.
When a person hits the ground at speed 2x,
he should take 2d of damage ? not 4d.
Therefore, we should feel free to discard the
concept that kinetic energy is linearly related
to falling damage.
Thephysics falling damage system
All systems that purport to do something
useful usually begin with at least one assumption.
For the physics falling damage
system, I have made two assumptions:
First, I assume that velocity and damage
are linearly related. This is a good assumption,
because it is intuitively correct. Second,
I assume that maximum damage
occurs at terminal velocity. At best, this is
not an assumption at all, but fact. Indeed,
where else could maximum damage occur?
Not when the speed of the object is still
increasing at a significant rate ? that is,
before terminal velocity is reached. And
certainly, any additional damage due to
wind burn after terminal velocity is reached
(for those falling extraordinary distances)
does not come under the heading of falling
damage.
In the AD&D game, maximum falling
damage is 20d6. We do not intend to
change this. Earlier, we established that the
terminal velocity of an adventurer?s fall was
reached at a distance of 256 feet. Thus, we
know that 20d6 of damage must be incurred
after a fall of 256 feet. With this knowledge,
and the physics equations from the accompanying
article, we can devise a new system
for falling damage.
Our starting point is equation 8, which
relates velocity to acceleration and the
distance fallen. If we look at Table I, we see
that the instantaneous velocity for the first
second is 32 ft./sec. If we substitute this
quantity for the acceleration due to gravity
(ag) in equation 8 from the other article, we
can derive this equation:
![]()
Unfortunately, the equation above does
not include the effect of air resistance on the
velocity of a falling body, but the mathematics
of determining air resistance are far too
complicated to be treated here. Fortunately,
however, air resistance only causes Vz (the
velocity in the ?down? direction) to be
reduced by a few feet per second; therefore,
we can consider it negligible in our calculations.
Ironically, the very force that makes
the physics falling damage system possible
(by creating terminal velocity) contributes
very little to the determination of velocity
itself. And velocity is the factor we need to
measure to determine falling damage.
From Table I, we know that at a distance
fallen of 256 feet, the speed of the falling
person is 128 ft./sec. We also know that the
damage is 20d6, because the body reaches
terminal velocity at 256 feet. We can plug
this information into the preceding equation
to determine the dice of damage per distance
fallen:
![]()
For an initial velocity (V0) of zero this,
equation reduces to:
128 ft./sec. = k x 20d6
Dividing 128 ft./sec. by 20d6 yields the
value of the constant:
(128 ft./sec.) / 20d6 = 6.4 ft./(sec. x d6)
Using d as the symbol for dice of damage,
we can rewrite the main equation as:
![]()
We can solve the equation for d by dividing
the right side of the expression by 6.4:
![]()
Again, since the initial speed of the falling
body is usually zero, we can simplify the
equation further to:
![]()
Now we have a simple equation
determines falling damage:
Dice of damage (d) equals 5 times the
square root of the distance fallen (z),
divided by 4.
Rounding out the system: saving throws
Although the equation above has all the
elements we need to determine damage, the
physics system is not yet complete. Let's
make a simple check on the new system as it
stands, and see if the results are reasonable.
If we use 10 feet for z (the distance fallen),
the result is 3.95 dice of damage. Indeed,
that seems to be quite a bit of damage for a
mere 10-foot drop (unless the character
lands on his head). Both of the old systems
would inflict only 1d6 for a 10-foot fall ?
significantly less than our new system. But
as I said, the physics system is not yet complete,
and to finish it, we must solve this
problem.
The solution lies on page 81 of the
DMG,
where falling damage
is described as an attack form that allows
the victim a bonus on his saving throw if
he's wearing magic armor. A saving throw
for falling damage? Never has such a thing
existed in the AD&D rules. Thus,
we have
the opportunity to fix two holes at once: one
in the game itself, and one in our new damage
system.
The saving throw for the physics system
is based on dexterity. (Estimate the dexterity
of monsters based on their physical
characteristics and other attributes.) Only
those who can maneuver in some way can
obtain a saving throw. For instance, neither
a bird bereft of its wings nor a man whose
legs and arms are bound can save against
falling damage. (However, if the man?s legs
are bound while his arms are free, he saves
at -10. If his arms are bound while his legs
are free, he saves at -5.)
If the distance fallen is more than 2 feet,
you must make a subtraction from the
character?s dexterity to determine the saving
throw. This subtraction equals the
number of damage dice done to the creature,
reduced to an integer (see the tables
that follow). For instance, for a 10-foot fall,
the character takes 3d6+ of damage, so the
character must subtract 3 from his dexterity
score and use that number as a basis for the
saving throw.
In the physics system, three saving
throws are possible.
* The first throw determines
whether only half damage is incurred
from the fall: roll the character's adjusted
dexterity score or less on 1d20 for success.
Also use the adjusted dexterity score to
determine the other two saves.
** If the first
roll is successful, a second save is possible: a
roll of one-half of the first number (round
down) or less on 1d20 means the victim
takes one-fourth normal damage.
*** Finally, if the first and second rolls are made, a third
save is possible: if this roll equals one-fourth
of the base number or less, the character
receives one-eighth damage. The damage
cannot be reduced further, and a minimum
of one point of damage is mandatory.
Our first example of the system in action
(3.94 damage dice for a 10-foot fall) shows
that the new system?s equation may yield a
remainder; and in fact, this is usually the
case. To translate this leftover fraction into
an equivalent amount of damage, round off
the remainder to the nearest hundredth and
use these guidelines: a fraction of .16 or less
equals 1 point of damage; .17 through .33
equals 1-2 points of damage; .34 through
.50 equals 1-3 points; a fraction of .51
through .75 equals 1-4 points; and .76
through .99 equals 1-5 points. For example,
after a fall of 10 feet the victim takes 3d6
plus 1-5 points of damage (before considering
saving throws), since the remainder of
.94 calls for an extra 1-5 points to be added
to the total.
As a final note, the reader should realize
that this system tends to break down at
distances very close to the ground. For falls
of 2 feet or less, only 1 point of damage is
incurred. Falls of between 2 feet and 5 feet,
including falls from horseback, should cause
1d6 of damage.
The following examples illustrate how the
physics falling damage system works as a
whole. The second example includes calculations
for falls in which the character starts
with a velocity above zero ? for instance,
when the character is thrown by a giant.
Example one
A thief with 33 hit points and a dexterity
score of 19 falls from a height of 170 feet.
According to the formula, the fall does
16.29 dice of damage, which converts to
16d6 and 1-2 extra points. To compute the
thief's saving throws, first subtract 16 from
the character?s dexterity score (corresponding
to the 16d6), so a 3 or less must be
rolled for the first saving throw. Miraculously,
the roll is a 3, so the thief suffers
?only? half damage. Since the first save
was successful, the thief can try for the
second saving throw. Because half of 3 is
1.5, the save to obtain one-quarter damage
is a roll of 1 on 1d20 (all fractions are
rounded down in saving-throw calculations).
This time, the roll is a 2, so the thief
barely misses the second save. No further
saving throw is possible, so the thief takes
half damage from the fall.
After the saving-throw procedure is completed,
we determine the actual damage. A
roll of 16d6 generates a result of 66, and a 1
is rolled for the additional 1-2 points possible,
so the total full damage is 67 points.
Since the character made his first save, he
takes only half of that amount, or 34 hit
points of damage. (When calculating damage,
round fractions up.) The thief, who
started with 33 hit points, now has -1 hit
points and is quite possibly going to succumb
to his injuries.
Armor modifications: Now, let?s back
up
for a minute. What if the thief was wearing
+1 leather armor? The bonus for his magic
armor is added to his saving throw requirement,
meaning that his first saving throw
for half damage must be 4 or less (instead of
3). The requirements for his subsequent
saving throws are based on the new
amount; now, the character must roll 2 or
less for one-quarter damage (instead of 1),
and 1 or less for one-eighth damage. So if
the second roll was a 2, as we proposed in
first part of this example, the thief would
take one-quarter damage and would still be
relatively healthy. In addition, the thief now
gets to try the third throw for one-eighth
damage. Even if the result of this third roll
is not a 1, he now only takes one-quarter
damage, which means 17 hit points. Because
he was wearing the +1 leather armor,
he still has 16 hit points left.
Example two
Now let?s see what happens if a 12-foottall
stone giant picks up the thief
and hurls
him off a 90-foot-high cliff. To determine
the damage in this case, we cannot use the
simple equation that we used for example
one. We must consider the extra speed
imparted to the thief when the giant hurls
him downward. To do this, we have to back
up one step to the equation containing the
expression (V0 / 6.4) and plug in a value for
V0 that is not zero:
![]()
Let's assume that the giant can impart a
speed of 20 miles per hour to the thief's
body. By multiplying the speed by 5280 (the
number of feet in a mile) and dividing by
3600 (the number of seconds in an hour),
we can convert this speed into the proper
units for the equation, feet per second. The
result is 29.3 ft./sec.
The thief falls 90 feet (the height of the
cliff) plus 12 feet, assuming the giant throws
the thief by lifting him over his head and
then casting him downward. Therefore:
![]()
This figure converts to 17d6 plus 1-2
points of damage.
The thief is worse off than he was in
example one, even though he?s falling a
shorter distance. Velocity is the key to this
system, and the giant has substantially
increased the velocity of the thief's fall, so
more damage dice are rolled. A modifier of
-17 is added to the thief's dexterity to determine
the first saving throw, compared to
-16 from the previous example. The thief
needs a roll of 2 or less to receive half damage,
and (assuming that roll succeeds) a roll
of 1 to take only one-quarter damage. He
has no chance of decreasing his damage to
one-eighth of the base amount, since half of
1 (rounded down) is zero, and a roll of zero
is not possible.
We can use the same rolls from example 1
for comparison. For the first roll, the thief
gets a 3. But in this case, the thief needs a 2
or less, so he takes full damage and has no
chance for further reductions. Let?s say that
the roll of 17d6 generates 68 points of damage,
and 1 point is determined for the extra
1-2 points possible. That makes a total of 69
hit points of damage. The thief is quite dead
at -36 hit points.
Armor modifications: Again, let?s use the
same modification from example one. The
thief wears +1 leather armor. Therefore, his
first saving throw is 3 instead of 2. Since a 3
was rolled, the thief receives only half damage.
The next roll is a 2, but the thief
needed a 1 to reduce the damage to onequarter.
Thus, he only makes the first
throw, and the thief takes half damage. Half
of 69 points is 35 (remember ? round up
for damage, down for saving throws). After
the fall, the thief has -2 hit points and is still
in pretty bad shape, although he might
survive under the right circumstances (for
instance, if someone happened to be close to
his point of impact and could administer
immediate aid).
The following chart shows the damage
caused by falls from distances of 10 feet
through 260 feet, at 10? intervals, assuming
an initial velocity of zero. To figure the
damage for intermediate distances, plug the
proper distance (z) into the equation.
The column marked ?old systems? lists
the PH version first, followed
by the cumulative system from
DRAGON® issue #70.
| Distance
(ft.) |
Damage,
new system |
Damage,
old systems <PH> |
- <WSG> |
| 10 | 3d6 + 1-5 | 1d6 | 1d6 |
| 20 | 5d6 + 1-4 | 2d6 | 3d6 |
| 30 | 6d6 + 1-5 | 3d6 | 6d6 |
| 40 | 7d6 + 1-5 | 4d6 | 10d6 |
| 50 | 8d6 + 1-5 | 5d6 | 15d6 |
| 60 | 9d6 + 1-4 | 6d6 | 20d6 |
| 70 | 10d6 + 1-3 | 7d6 " | - |
| 80 | 11d6 + 1 | 8d6 " | - |
| 90 | 11d6 + 1-5 " | 9d6 | - |
| 100 | 12d6 + 1-3 " | 10d6 | - |
| 110 | 13d6 + 1 " | 11d6 | - |
| 120 | 13d6 + 1-4 " | 12d6 | - |
| 130 | 14d6 + 1-2 " | 13d6 | - |
| 140 | 14d6 + 1-5 " | 14d6 | - |
| 150 | 15d6 + 1-2 " | 15d6 | - |
| 160 | 15d6 + 1-5 | 16d6 " | - |
| 170 | 16d6 + 1-2 " | 17d6 | - |
| 180 | 16d6 + 1-5 " | 18d6 | - |
| 190 | 17d6 + 1-2 " | 19d6 | - |
| 200 | 17d6 + 1-4 " | 20d6 | - |
| 210 | 18d6 + 1 " | " | - |
| 220 | 18d6 + 1-4 " | " | - |
| 230 | 18d6 + 1-5 " | " | - |
| 240 | 19d6 + 1-3 | " | - |
| 250 | 19d6 + 1-5 | " | - |
| 260 | 20d6 | " | - |
<like all #s in the article, double check the above, *2wice*>
Kinetic energy is the key
A brief rebuttal to the physics falling damage system
by Steven Winter
While it seems intuitively correct that
injuries suffered in a fall are linearly related
to velocity, that notion is incorrect. The
factor that Ms. Parker rejects ? kinetic
energy -- is the real culprit.
The confusion arises from the fact that
the collision between a falling body and the
earth is essentially inelastic. In an elastic
collision, two bodies smash together and
then bounce apart; colliding billiard balls
are a classic example. Kinetic energy is
conserved in an elastic collision. In an
inelastic collision, two bodies smash together
and stick together, like coupling
railroad cars. When a body falls to the
earth, it doesn't bounce (at least not much);
gravity pins it to the surface. Kinetic energy
is not conserved in an inelastic collision,
contrary to what Ms. Parker's example
illustrates. Momentum is conserved, and
total energy is conserved, but kinetic energy
is not. In the case of a human body hitting
the earth, the amount of kinetic energy
conserved as actual kinetic energy is astoundingly
small -- much less than 0.00001
percent (if we ignore the earth?s kinetic
energy, assuming its velocity to be zero).
What happens to the kinetic energy?
Some of it produces a loud "thud," some of
it raises a cloud of dust, and some of it
produces heat. But most of it becomes
"internal energy" that dissipates by doing
work: breaking bones, crushing organs, and
setting up elastic waves in the body and in
the earth. I couldn't state offhand how
much energy is absorbed by the ground and
how much by the person, but most of it
probably goes into the person, since a human
body is more elastic than packed earth.
The important point is that injuries are
caused by this "missing" kinetic energy,
which is proportional to the square of the
falling body's velocity, or to some constant
fraction of the square of the velocity.
How this system plays
As might be expected, relating damage to
kinetic energy has an interesting effect on
the game.
The table below is an analysis of the
energy states of a falling body. D is the
distance that the object has fallen, measured
in feet. T is the number of seconds that the
object has been in free fall. V is the object?s
velocity at distance D, in feet per second.
V2 is the square of the velocity, and is directly
proportional to the object's kinetic
energy. is the increase in V2 from the
previous entry for D and the current value
of D, a number which is directly proportional
to the increase in the object?s kinetic
energy.
What the table shows is that, when a
falling object's kinetic energy is sampled at
regular intervals of distance, the increase is
linear. If this constant increase is arbitrarily
assumed to equal 1d6 points of damage for
every 10 feet fallen, we are right back at the
game's original falling damage system,
as
expressed in the PH. Possibly
a coincidence, but . . .
| D | T | V | V <> | 2 <> |
| 10 | 0.79 | 25.29 | 640 | 640 |
| 20 | 1.11 | 35.77 | 1,280 | 640 |
| 30 | 1.36 | 43.81 | 1,920 | 640 |
| 40 | 1.58 | 50.59 | 2,560 | 640 |
| 50 | 1.76 | 56.56 | 3,200 | 640 |
| 60 | 1.93 | 61.96 | 3,840 | 640 |
| 70 | 2.0 9 | 66.93 | 4,480 | 640 |
| 80 | 2.23 | 71.55 | 5,120 | 640 |
| 90 | 2.37 | 75.89 | 5,760 | 640 |
| 100 | 2.50 | 80.00 | 6,400 | 640 |
| 150 | 3.06 | 97.97 | 9,600 | 3,200 |
| 200 | 3.53 | 113.13 | 12,800 | 3,200 |
D
A
M
A
G
E
by Frank Mentzer
I must preface this brief article with a statement
on Officiality. Please note that game-related information
appearing in this magazine, under the
name of any author, is presented for the consideration
of the Gentle Readers, and feedback is
definitely encouraged. The regular columns by E.
Gary Gygax are, indeed, Official, but are not
Final. You who are now reading these lines can
affect the course of AD&D™ rules,
by responding
with your comments, both good and bad, on the
information offered. The Final versions of the
spells, character classes, and other Official tidbits
from Gary will be published separately in the
future. We will definitely release a new hardback
book, the AD&D Expansion volume,
within a few
score fortnights. It will, it is hoped, contain details
to explain and correct all the little holes in the
system that we can find, along with vast amounts
of new information. And by the way, Monster
Manual Vol. 2 is already in production,
and will
appear this year, long before the Expansion
volume. And MM2 looks so good
that I won’t say
another word about it.
As to the problem with falling damage — well, it
all started back in the early 1970’s. (Editor’s note:
The problem came to light during the preparation
of Mr. Gygax’s column on the Thief-Acrobat
“split class,” which appeared in issue #69 of
DRAGON™ Magazine. It was addressed in a brief
note published along with that article, with a
promise that a more detailed explanation — this
article — would be forthcoming.)
Gary has always used a geometrically increasing
system for falling damage in AD&D
games;
the trouble arose because that system simply
never made it into the rule books.
When the AD&D PH was
being
assembled, a brief section on falling
damage was
included: a mere 7½ lines that offers more advice
on broken bones and sprains than on falling
damage. As we now understand the event, the
section was not included in the first draft, and the
editors requested a brief insert on this frequently
referred-to topic. So Gary hastily wrote a sentence
describing damage as “1d6 per 10’ for each
10’ fallen.” Someone removed the “per 10’” as
being (so it was thought) redundant, and off we
went. That section was later quoted
in passing in
the Aerial Adventures section of the DMG, thereby becoming further
entrenched <>
in our game procedures.
The main point of current controversy seems to
be the simple fact that everyone has been using
“1d6 for each 10’ fallen” for years, and the social
inertia of Custom is still being cited as a reason to
override common sense. And everyone still wants
to know if you get a saving throw against falling
damage; items do. (Note, however, that the “fall”
category on DMG p. 80 mentions specifically falls
of 5’ or so; in other words, a dropped item, rather
than one that sustains a long fall. Adjustments
should be applied for descents of greater distance
than 5’.) <(also: cf. Magic Armor
&& Saving Throws, DMG)>
Please understand first that when Gary writes
something, he assumes that no important changes
are being made between manuscript and printed
product. (I’m sure most, if not all, other accomplished,
best-selling authors assume the same
thing.) So Gary checks the overall look before we
in the TSR word factory send the final product off
to the printer — but he doesn’t review all the
details. After all, he knows what he wants to do in
his home-style games, and he writes more generalized,
system-applicable details for us. He
doesn’t play AD&D games strictly
by the book,
and usually has no reason to double-check details
in the books and other products before they are
sent out for printing. (I usually end up with those
tasks these days.)
So it was not until years after that first appearance
of the “1d6 per 10’ fallen” rule that Gary
finally noticed we’ve been doing it wrong all these
years.
The “correct” damage incurred by falling is 1d6
for the first 10’, 2d6 for the second 10’ (total 3d6
for a 20’ fall), 3d6 for the third 10’, and so on,
cumulative. The falling body reaches that 20d6
maximum shortly before passing the 60’ mark.
This is definitely more realistic than a straight 1d6
per 10’; using the latter rule, I’ve seen a tough
fighter dive off a cliff into a deep canyon, get up
and dust himself off, and then start chasing
monsters. If the DM had added, say, a saving
throw vs. death with a -12 penalty, that would
have been fairer; but there are no guidelines for
such a save, and DMs are free to encourage fantastic
stunts by ignoring inertia, the effect of
acceleration on a falling body, and other “real”
principles that apply in the “fantasy” world.
Note that the 1d6 is appropriate in the first
place because the gravity of Oerth (Gary’s
“world”) approximates that of our own planet,
which accelerates a falling body by 32 feet per
second for each second of fall. This would not be
the case for worlds with weaker gravities, where
1d4 might suffice, or some other method might be
used. In any case, however, the nature of gravity
is such that you speed up as you go: a 20’ fall
should be more than twice as damaging as a 10’
fall.
This cumulative system obviously makes pits a
lot nastier, and monks, thief-acrobats, and fly and
feather fall spells a lot more
useful.
Personally, I’d tend to be as tough as Gary’s
old/newly announced system, and then some.
Add a system shock roll for any falls of 60’ or
more (the “break point” for maximum damage),
plus a save vs. death for similarly long falls, to see
if you broke your neck or something. I might even
want to modify that saving throw — say, a penalty
of -1 for each 10’ fallen (not cumulative), but +1
per character level. (This isn’t Official, it’s opinion,
and this opinion continues:)
For saving throws, the cumulative system is so
much more deadly that I’d allow a saving throw
vs. death, for half damage if successful. But in
turn, I wouldn’t stop accumulating damage after
60’. The 20d6 maximum would still apply, but
after the “real” damage is totaled. For example, a
60’ fall inflicts 21d6 damage (save for half); a 70’
fall, 28d6 (save for 14d6, or half, damage); an 80’
fall, 36d6 (18d6 if saved against), and so forth.
The 20d6 maximum should apply to the net
effect, used if the save is failed in the examples
above, instead of the rolls of 21d6, 28d6, or 36d6
mentioned.
Write! Tell us what you’ve been doing for falling
damage, and what you think of the more realistic
system recently offered. Gary does read your
input — many of you have received replies from
the Good Sage by now — and he’ll clean up this
debated area soon.
FALLING DAMAGE (Added to original)
| Distance | Damage | - | - |
| 10 | 1d6 | - | - |
| 20 | 3d6 | - | - |
| 30 | 6d6 | - | - |
| 40 | 10d6 | - | - |
| 50 | 15d6 | - | - |
| 60 | 20d6 | System shock roll + save vs. death1 at -6, for half damage | - |
| 70 | - | System shock roll + save vs. death1 at -7, for half damage | - |
| 80 | - | System shock roll + save vs. death1 at -8, for half damage | - |
| 90 | - | System shock roll + save vs. death1 at -9, for half damage | - |
| 100 | - | System shock roll + save vs. death1 at -10, for half damage | - |
1 Very important: remember to modify this save
vs. death by +1 per character level.
Character could be interpreted as highest + # of
classes for multi-classed characters)
<check, finish>
Falling followup
Dear Dragon:
After reading the article on falling damage
in issue #70, I feel it is imperative that the
truth
be known about actual falling rates using the
laws of physics of the real world.
According to these laws, a falling body
accelerates geometrically. This is the foundation
of the “true” system, not that found
in the
Players Handbook. However, a look at the
speeds of a falling body during the first four
seconds after dropping from rest shows some
interesting results:
| Time (sec.) | Velocity
(ft/sec.) |
Distance fallen (ft.) |
| 1 | 32 | 16 |
| 2 | 64 | 64 |
| 3 | 96 | 144 |
| 4 | 128 | 256 |
Lo and behold! The object falls 48 feet during
the 2nd second — three times as far as
during the 1st second — yet its speed only
doubles. During the entire four seconds of
falling, the distance fallen per second increased
by a factor of 16, while the velocity of
the body only went up 4 times. The relationship
of distance to speed isn’t a geometric
progression, but a geometric retrogression!
Put in simple terms, after a fall of 64 feet, an
object strikes the ground twice as fast, and
presumably takes twice as much damage,
than it does after a 16-foot fall. In the Players
Handbook system, the object
would take 4
times more damage — a little high, but bearable.
However, in the “true” system put forth
in #70, damage would increase by 10 times!
This is not bearable.
On top of this, a falling object has a terminal
velocity — a speed at which, due to air friction,
the object will not continue to gain
speed. Thus, it should not strike any harder,
or take any more damage, for any increase in
the distance of the fall beyond the point where
the body reaches terminal velocity. In the real
world, this speed is reached at roughly 250
feet. In the Players Handbook system, damage
and (presumably) speed top out at 200
feet — again, not correct but bearable. In the
“true” system, it tops out at slightly under 60
feet. This is not acceptable at all!
Therefore, in light of the facts, the
accidental
system given in the Players Handbook
should be used, and the system that was
really intended should be scratched.
Scott D. Hoffrage
Miller Place, N.Y.
(Dragon #72)